toreeco.blogg.se

1. #Inclined plane physics calculator plus#
2. #Inclined plane physics calculator download#
3. #Inclined plane physics calculator free#

#Inclined plane physics calculator free#

We’re now ready to plug this value in for 𝑎. So when we plug in 𝑣 sub 𝑓 and 𝑡, we see that 𝑎 is negative 21 metres per second divided by 100 seconds or negative 0.21 metres per second squared. All this happens over the course of a time of 100 seconds. So our initial velocity is zero and that it attains a final velocity 𝑣 sub 𝑓 of 21 metres per second. We’re told that our car starts out from rest. But what about the acceleration 𝑎? We can recall that acceleration is defined as a change in velocity over change in time. We know the gravitational acceleration 𝑔 and we also know the sine of 𝜃.

#Inclined plane physics calculator plus#

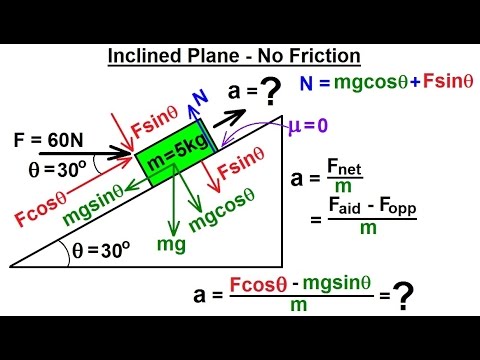
So it reads 𝐹 sub 𝑓 over 𝑚 is equal to 𝑔 sine 𝜃 plus 𝑎. If we then divide both sides of our equation by the mass of the car 𝑚, that term cancels out of two of our three terms and we now have 𝐹 sub 𝑓 over 𝑚 in the far left, which is what we want to solve for. This means when we apply Newton’s second law to forces in the 𝑥-direction, we can write 𝐹 sub 𝑓 minus 𝑚𝑔 times the sine of 𝜃 is equal to 𝑚 times 𝑎. If we divide our weight force 𝑚𝑔 into 𝑦- and 𝑥-components, then the right triangle formed has an angle of 𝜃 in its upper corner. Two of our three forces in our diagram act along this axis 𝐹 sub 𝑓 and a component of the weight force 𝑚𝑔. Let’s consider the forces in the 𝑥-direction. If we place a pair of coordinate axes on our diagram so that 𝑥 is acting up the hill and 𝑦 is positive perpendicularly to the plane, then we become able to apply the second law to both directions. The second law says that the net force acting on an object is equal to that object’s mass multiplied by its acceleration. To solve for that force per ton of the car’s mass, let’s begin by recalling Newton’s second law of motion. And finally, there is a resistive force due to friction, which we’ve called 𝐹 sub 𝑓, acting on the car and opposing its downward motion. There is also a normal force pushing the car perpendicularly away from the plane of the hill. That’s equal to the mass of the car times the acceleration due to gravity 𝑔, which we’re told to treat as exactly 9.8 metres per second squared. Example: at a length of 10 and a height of 5 meters, the angle of slope is 30, the velocity of a ball is 7.3824 m/s and the time to get there is 2.7091 seconds. The velocity is the speed of the object at the length l. First, there is the force of gravity acting down. Calculation of duration and velocity of an object rolling down a slope ( inclined plane ). To start on our solution, let’s draw in the forces that are acting on the car while it descends. Knowing this about the car’s descent, we want to solve for the resistive force - we’ve called it 𝐹 sub 𝑓 - applied to the car per ton of its mass 𝑚. After a time of 100 seconds, the car has achieved a particular speed 21 metres per second downhill. At 𝑡 equals zero, the car is released and begins to slide downhill.

#Inclined plane physics calculator download#

We’re told that we begin with a car at rest on a hillside inclined at an angle 𝜃 from the horizontal. Instructions Before viewing an episode, download and print the note-taking guides, worksheets, and lab data sheets for that episode, keeping the printed. Let’s begin our solution by drawing a sketch of this scenario. We want to calculate the resistance per ton of the car’s mass.

We’re told that the sine of the angle of inclination of the hill on which the car rests is one-half. And the speed it achieves after that time 21 metres per second we’ll call 𝑣 sub 𝑓.

We can call the elapsed time during which the car is speeding up 100 seconds 𝑡. Take 𝑔 to equal 9.8 metres per second squared.

Calculate the resistance per ton of the car’s mass. After 100 seconds, its velocity was 21 metres per second. A car of mass 𝑚 tons was initially at rest on a hill inclined at an angle 𝜃 to the horizontal, where sine of 𝜃 equals one-half.